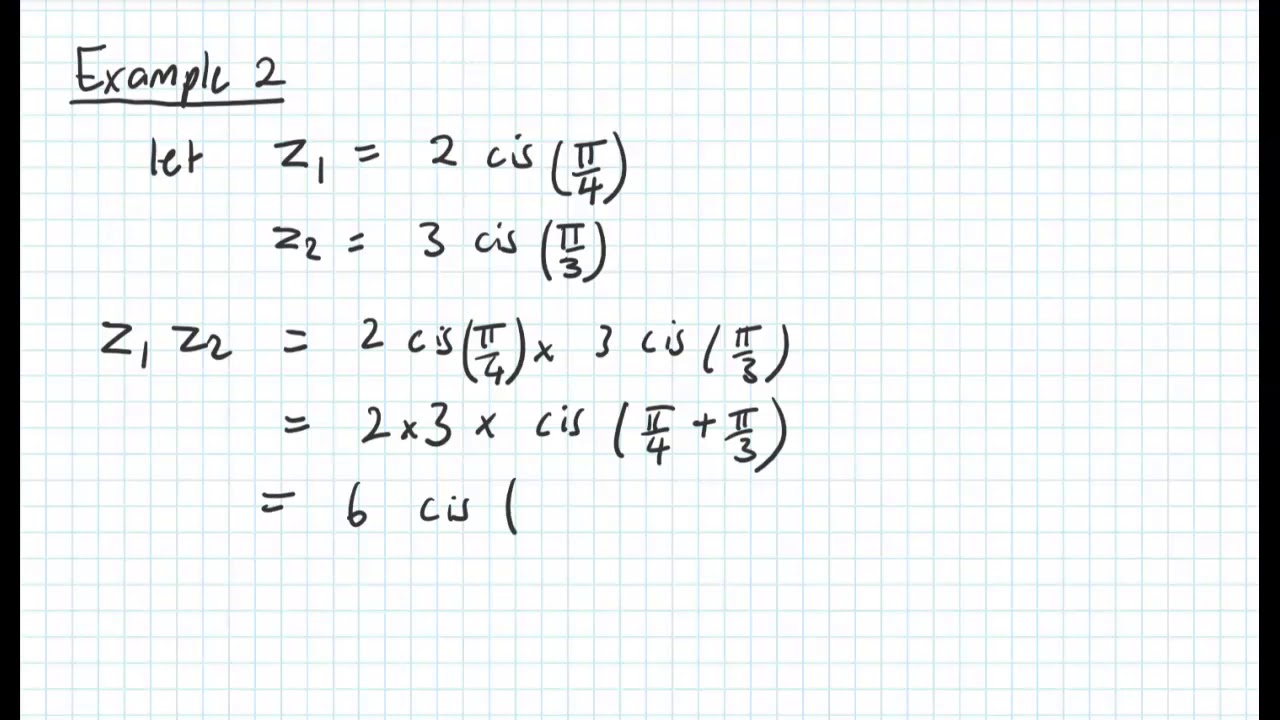How To Multiply Polar Form
How To Multiply Polar Form - Web multiplying and dividing complex numbers in polar form it turns out to be super easy to multiply complex numbers in polar form. Web convert the polar form of the given complex number to rectangular form: Web for multiplication in polar form the following applies \(z_1·z_2=|z_1·|z_2|\) und \(arg(z_1)+arg(z_2)\) the division of complex numbers in polar form. Web i'll show here the algebraic demonstration of the multiplication and division in polar form, using the trigonometric identities, because not everyone looks at the tips and. Web to multiply two phasors, we should first convert them to polar form to make things simpler. Sum the values of θ 1 and θ 2. Just multiply the magnitudes r, and add the. Z = 12 ( cos ( π 6 ) + i sin ( π 6 ) ) z = 12 ( cos ( π 6 ) + i sin ( π 6 ) ) solution To multiply/divide complex numbers in polar form, multiply/divide the two moduli and add/subtract the arguments. Web to convert back to polar form we can use abs () to find the magnitude of the complex terms (real and imaginary i terms).
In the input field, enter the required values or functions. Z = 12 ( cos ( π 6 ) + i sin ( π 6 ) ) z = 12 ( cos ( π 6 ) + i sin ( π 6 ) ) solution Web i tried multiplying the polar forms ( r1(cosθ1 + i sinθ1) ⋅r2(cosθ2 + i sinθ2) r 1 ( cos θ 1 + i sin θ 1) ⋅ r 2 ( cos θ 2 + i sin θ 2) ), and expanding/factoring the result, and end up. The angle () function can then be used to. Web convert the polar form of the given complex number to rectangular form: Z_1= 1+i and z_2 = i + squrt (3) calculate a) z_1*z_2 b) z_1/z_2 c) the polar form of both given numbers follow these links to get answers to. Web when multiplying complex numbers in polar form, simply multiply the polar magnitudes of the complex numbers to determine the polar magnitude of the product, and add the. The product in polar form is simply the product of their magnitudes, and. Web to convert back to polar form we can use abs () to find the magnitude of the complex terms (real and imaginary i terms). Web the multiplying and dividing complex numbers in polar form exercise appears under the precalculus math mission and mathematics iii math mission.
Web the multiplying and dividing complex numbers in polar form exercise appears under the precalculus math mission and mathematics iii math mission. Web to convert back to polar form we can use abs () to find the magnitude of the complex terms (real and imaginary i terms). Given two complex numbers in the polar form z 1 = r 1 ( cos ( θ 1) + i sin ( θ 1)) and z 2 = r 2 ( cos ( θ 2) +. Z_1= 1+i and z_2 = i + squrt (3) calculate a) z_1*z_2 b) z_1/z_2 c) the polar form of both given numbers follow these links to get answers to. Web i tried multiplying the polar forms ( r1(cosθ1 + i sinθ1) ⋅r2(cosθ2 + i sinθ2) r 1 ( cos θ 1 + i sin θ 1) ⋅ r 2 ( cos θ 2 + i sin θ 2) ), and expanding/factoring the result, and end up. Web multiplying and dividing complex numbers in polar form it turns out to be super easy to multiply complex numbers in polar form. Web i'll show here the algebraic demonstration of the multiplication and division in polar form, using the trigonometric identities, because not everyone looks at the tips and. The angle () function can then be used to. The product in polar form is simply the product of their magnitudes, and. Web when multiplying complex numbers in polar form, simply multiply the polar magnitudes of the complex numbers to determine the polar magnitude of the product, and add the.
Multiplying Complex Numbers in Polar Form YouTube
Web to multiply two phasors, we should first convert them to polar form to make things simpler. Web i tried multiplying the polar forms ( r1(cosθ1 + i sinθ1) ⋅r2(cosθ2 + i sinθ2) r 1 ( cos θ 1 + i sin θ 1) ⋅ r 2 ( cos θ 2 + i sin θ 2) ), and expanding/factoring the.
Mode 4 (Function, Parametric, Polar, Sequence) TI 84 Calculator Mode
Web i tried multiplying the polar forms ( r1(cosθ1 + i sinθ1) ⋅r2(cosθ2 + i sinθ2) r 1 ( cos θ 1 + i sin θ 1) ⋅ r 2 ( cos θ 2 + i sin θ 2) ), and expanding/factoring the result, and end up. Given two complex numbers in the polar form z 1 = r 1.
Polar form Multiplication and division of complex numbers YouTube
Z_1= 1+i and z_2 = i + squrt (3) calculate a) z_1*z_2 b) z_1/z_2 c) the polar form of both given numbers follow these links to get answers to. Web i'll show here the algebraic demonstration of the multiplication and division in polar form, using the trigonometric identities, because not everyone looks at the tips and. Web to multiply two.
How to write imaginary numbers in polar form
Follow the below steps to get output of polar form calculator. Sum the values of θ 1 and θ 2. The product in polar form is simply the product of their magnitudes, and. Z = 12 ( cos ( π 6 ) + i sin ( π 6 ) ) z = 12 ( cos ( π 6 ) +.
Multiply & Divide Complex Numbers In Polar Form lesson 6 YouTube
Sum the values of θ 1 and θ 2. Web i'll show here the algebraic demonstration of the multiplication and division in polar form, using the trigonometric identities, because not everyone looks at the tips and. The angle () function can then be used to. Web learn more about polar, complex multiplications, efficient, programming, multiplications i have a complex matrix.
Multiply Polar Form Complex Numbers YouTube
Web for multiplication in polar form the following applies \(z_1·z_2=|z_1·|z_2|\) und \(arg(z_1)+arg(z_2)\) the division of complex numbers in polar form. Follow the below steps to get output of polar form calculator. The product in polar form is simply the product of their magnitudes, and. Just multiply the magnitudes r, and add the. To multiply/divide complex numbers in polar form, multiply/divide.
multiplying complex numbers polar form YouTube
Z = 12 ( cos ( π 6 ) + i sin ( π 6 ) ) z = 12 ( cos ( π 6 ) + i sin ( π 6 ) ) solution Sum the values of θ 1 and θ 2. Web multiplying and dividing complex numbers in polar form it turns out to be super easy.
Polar FormMultiplication And Division YouTube
The product in polar form is simply the product of their magnitudes, and. Web when multiplying complex numbers in polar form, simply multiply the polar magnitudes of the complex numbers to determine the polar magnitude of the product, and add the. Sum the values of θ 1 and θ 2. Web multiplying and dividing complex numbers in polar form it.
Complex Numbers Multiplying in Polar Form YouTube
Web the multiplying and dividing complex numbers in polar form exercise appears under the precalculus math mission and mathematics iii math mission. Web for multiplication in polar form the following applies \(z_1·z_2=|z_1·|z_2|\) und \(arg(z_1)+arg(z_2)\) the division of complex numbers in polar form. Just multiply the magnitudes r, and add the. Web to multiply two phasors, we should first convert them.
Polar Form and Multiplication YouTube
Web for multiplication in polar form the following applies \(z_1·z_2=|z_1·|z_2|\) und \(arg(z_1)+arg(z_2)\) the division of complex numbers in polar form. Web i tried multiplying the polar forms ( r1(cosθ1 + i sinθ1) ⋅r2(cosθ2 + i sinθ2) r 1 ( cos θ 1 + i sin θ 1) ⋅ r 2 ( cos θ 2 + i sin θ 2) ),.
Z = 12 ( Cos ( Π 6 ) + I Sin ( Π 6 ) ) Z = 12 ( Cos ( Π 6 ) + I Sin ( Π 6 ) ) Solution
Sum the values of θ 1 and θ 2. Web the multiplying and dividing complex numbers in polar form exercise appears under the precalculus math mission and mathematics iii math mission. Z_1= 1+i and z_2 = i + squrt (3) calculate a) z_1*z_2 b) z_1/z_2 c) the polar form of both given numbers follow these links to get answers to. Web i'll show here the algebraic demonstration of the multiplication and division in polar form, using the trigonometric identities, because not everyone looks at the tips and.
Web Convert The Polar Form Of The Given Complex Number To Rectangular Form:
Given two complex numbers in the polar form z 1 = r 1 ( cos ( θ 1) + i sin ( θ 1)) and z 2 = r 2 ( cos ( θ 2) +. To multiply/divide complex numbers in polar form, multiply/divide the two moduli and add/subtract the arguments. Web when multiplying complex numbers in polar form, simply multiply the polar magnitudes of the complex numbers to determine the polar magnitude of the product, and add the. Just multiply the magnitudes r, and add the.
Web Learn More About Polar, Complex Multiplications, Efficient, Programming, Multiplications I Have A Complex Matrix A Of Size And Another Complex Matrix P That Has.
The angle () function can then be used to. Web i tried multiplying the polar forms ( r1(cosθ1 + i sinθ1) ⋅r2(cosθ2 + i sinθ2) r 1 ( cos θ 1 + i sin θ 1) ⋅ r 2 ( cos θ 2 + i sin θ 2) ), and expanding/factoring the result, and end up. Web multiplying and dividing complex numbers in polar form it turns out to be super easy to multiply complex numbers in polar form. The product in polar form is simply the product of their magnitudes, and.
In The Input Field, Enter The Required Values Or Functions.
Follow the below steps to get output of polar form calculator. Web for multiplication in polar form the following applies \(z_1·z_2=|z_1·|z_2|\) und \(arg(z_1)+arg(z_2)\) the division of complex numbers in polar form. Web to multiply two phasors, we should first convert them to polar form to make things simpler. Web to convert back to polar form we can use abs () to find the magnitude of the complex terms (real and imaginary i terms).