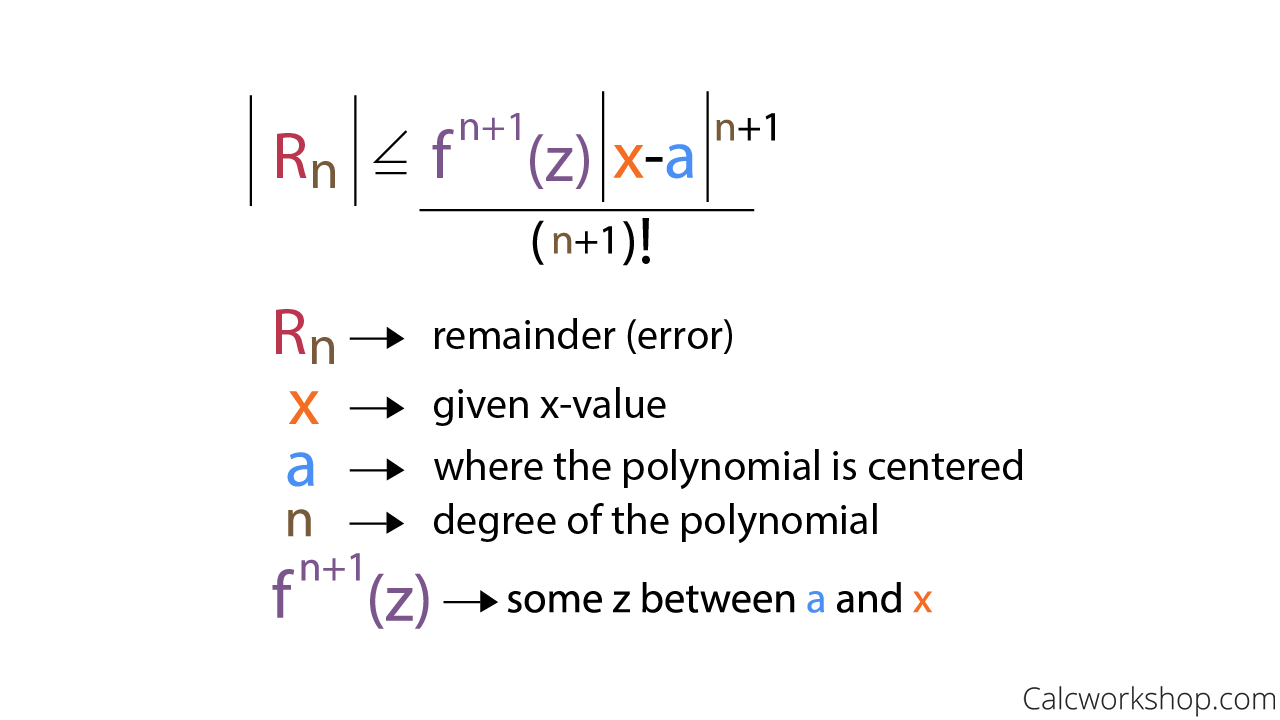Lagrange Form Of Remainder
Lagrange Form Of Remainder - By construction h(x) = 0: Web to compute the lagrange remainder we need to know the maximum of the absolute value of the 4th derivative of f on the interval from 0 to 1. Since the 4th derivative of ex is just. Consider the function h(t) = (f(t) np n(t))(x a)n+1 (f(x) p n(x))(t a) +1: Web now, the lagrange formula says |r 9(x)| = f(10)(c)x10 10! Recall this theorem says if f is continuous on [a;b], di erentiable on (a;b), and. Web differential (lagrange) form of the remainder to prove theorem1.1we will use rolle’s theorem. Also dk dtk (t a)n+1 is zero when. Now, we notice that the 10th derivative of ln(x+1), which is −9! The remainder r = f −tn satis es r(x0) = r′(x0) =:::
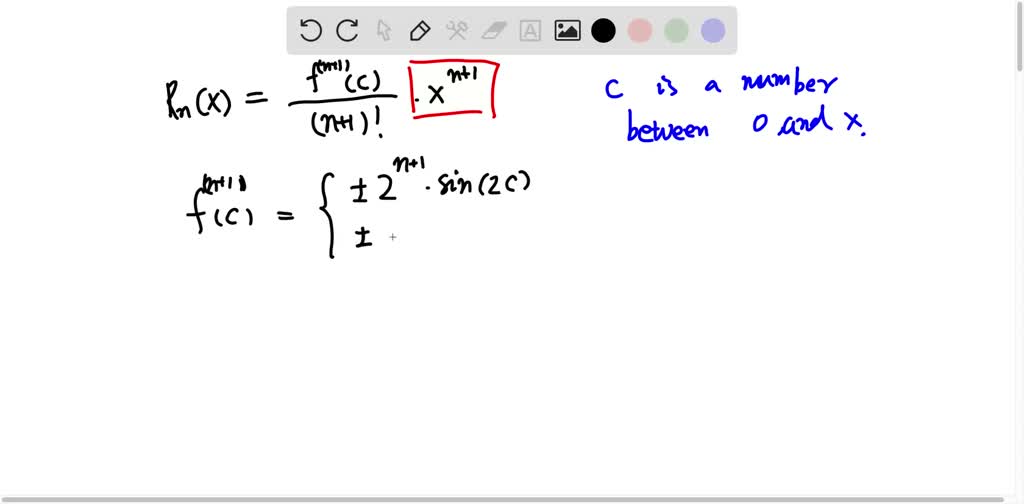
Web now, the lagrange formula says |r 9(x)| = f(10)(c)x10 10! For some c ∈ ( 0, x). Web the formula for the remainder term in theorem 4 is called lagrange’s form of the remainder term. Xn+1 r n = f n + 1 ( c) ( n + 1)! The cauchy remainder after terms of the taylor series for a. Watch this!mike and nicole mcmahon. Web the cauchy remainder is a different form of the remainder term than the lagrange remainder. Web what is the lagrange remainder for sin x sin x? Web note that the lagrange remainder r_n is also sometimes taken to refer to the remainder when terms up to the. Where c is between 0 and x = 0.1.
Recall this theorem says if f is continuous on [a;b], di erentiable on (a;b), and. The remainder r = f −tn satis es r(x0) = r′(x0) =::: X n + 1 and sin x =∑n=0∞ (−1)n (2n + 1)!x2n+1 sin x = ∑ n = 0 ∞ ( −. Web what is the lagrange remainder for sin x sin x? Notice that this expression is very similar to the terms in the taylor. F(n)(a + ϑ(x − a)) r n ( x) = ( x − a) n n! Now, we notice that the 10th derivative of ln(x+1), which is −9! The cauchy remainder after terms of the taylor series for a. When interpolating a given function f by a polynomial of degree k at the nodes we get the remainder which can be expressed as [6]. Since the 4th derivative of ex is just.
Answered What is an upper bound for ln(1.04)… bartleby
Web in my textbook the lagrange's remainder which is associated with the taylor's formula is defined as: Web remainder in lagrange interpolation formula. F ( n) ( a + ϑ ( x −. Web to compute the lagrange remainder we need to know the maximum of the absolute value of the 4th derivative of f on the interval from 0.
Solved Find the Lagrange form of remainder when (x) centered
Web remainder in lagrange interpolation formula. Web note that the lagrange remainder r_n is also sometimes taken to refer to the remainder when terms up to the. Now, we notice that the 10th derivative of ln(x+1), which is −9! X n + 1 and sin x =∑n=0∞ (−1)n (2n + 1)!x2n+1 sin x = ∑ n = 0 ∞ (.
Infinite Sequences and Series Formulas for the Remainder Term in
When interpolating a given function f by a polynomial of degree k at the nodes we get the remainder which can be expressed as [6]. Web now, the lagrange formula says |r 9(x)| = f(10)(c)x10 10! Notice that this expression is very similar to the terms in the taylor. Now, we notice that the 10th derivative of ln(x+1), which is.
SOLVEDWrite the remainder R_{n}(x) in Lagrange f…
Web the cauchy remainder is a different form of the remainder term than the lagrange remainder. X n + 1 and sin x =∑n=0∞ (−1)n (2n + 1)!x2n+1 sin x = ∑ n = 0 ∞ ( −. Consider the function h(t) = (f(t) np n(t))(x a)n+1 (f(x) p n(x))(t a) +1: Web differential (lagrange) form of the remainder to.
9.7 Lagrange Form of the Remainder YouTube
By construction h(x) = 0: Lagrange’s form of the remainder 5.e: (x−x0)n+1 is said to be in lagrange’s form. Since the 4th derivative of ex is just. Web the formula for the remainder term in theorem 4 is called lagrange’s form of the remainder term.
Lagrange Remainder and Taylor's Theorem YouTube
Now, we notice that the 10th derivative of ln(x+1), which is −9! Web note that the lagrange remainder r_n is also sometimes taken to refer to the remainder when terms up to the. When interpolating a given function f by a polynomial of degree k at the nodes we get the remainder which can be expressed as [6]. Web what.
Taylor's Remainder Theorem Finding the Remainder, Ex 1 YouTube
Web the stronger version of taylor's theorem (with lagrange remainder), as found in most books, is proved directly from the mean value theorem. Watch this!mike and nicole mcmahon. Since the 4th derivative of ex is just. Now, we notice that the 10th derivative of ln(x+1), which is −9! When interpolating a given function f by a polynomial of degree k.
Remembering the Lagrange form of the remainder for Taylor Polynomials
Web the remainder f(x)−tn(x) = f(n+1)(c) (n+1)! By construction h(x) = 0: The remainder r = f −tn satis es r(x0) = r′(x0) =::: Web note that the lagrange remainder r_n is also sometimes taken to refer to the remainder when terms up to the. Web need help with the lagrange form of the remainder?
Lagrange form of the remainder YouTube
For some c ∈ ( 0, x). Web the remainder f(x)−tn(x) = f(n+1)(c) (n+1)! Web in my textbook the lagrange's remainder which is associated with the taylor's formula is defined as: That this is not the best approach. F(n)(a + ϑ(x − a)) r n ( x) = ( x − a) n n!
Solved Find the Lagrange form of the remainder Rn for f(x) =
Since the 4th derivative of ex is just. Also dk dtk (t a)n+1 is zero when. By construction h(x) = 0: Web remainder in lagrange interpolation formula. Web the remainder f(x)−tn(x) = f(n+1)(c) (n+1)!
F ( N) ( A + Θ ( X −.
Web differential (lagrange) form of the remainder to prove theorem1.1we will use rolle’s theorem. Since the 4th derivative of ex is just. Where c is between 0 and x = 0.1. When interpolating a given function f by a polynomial of degree k at the nodes we get the remainder which can be expressed as [6].
Now, We Notice That The 10Th Derivative Of Ln(X+1), Which Is −9!
Web now, the lagrange formula says |r 9(x)| = f(10)(c)x10 10! Web note that the lagrange remainder r_n is also sometimes taken to refer to the remainder when terms up to the. F(n)(a + ϑ(x − a)) r n ( x) = ( x − a) n n! Web in my textbook the lagrange's remainder which is associated with the taylor's formula is defined as:
That This Is Not The Best Approach.
X n + 1 and sin x =∑n=0∞ (−1)n (2n + 1)!x2n+1 sin x = ∑ n = 0 ∞ ( −. Also dk dtk (t a)n+1 is zero when. Web the formula for the remainder term in theorem 4 is called lagrange’s form of the remainder term. Watch this!mike and nicole mcmahon.
For Some C ∈ ( 0, X).
By construction h(x) = 0: Notice that this expression is very similar to the terms in the taylor. Consider the function h(t) = (f(t) np n(t))(x a)n+1 (f(x) p n(x))(t a) +1: Web the stronger version of taylor's theorem (with lagrange remainder), as found in most books, is proved directly from the mean value theorem.